Is the new Pa. House map better for Democrats or Republicans? We tested it.
The new map will help determine each party’s prospects for winning a majority — and with it the power to make policy affecting millions of Pennsylvanians.
Officials proposed a new map Thursday for Pennsylvania’s state House districts, kicking off a redistricting process that will shape power in the state for the next decade — and one that’s already sparking political fights along the way.
The House, like the state Senate, is currently dominated by Republicans, and Democrats have long hoped to win back control after decades of mostly GOP rule. The new map proposed Thursday will help determine each party’s prospects for winning a majority — and with it the power to make policy affecting the lives of millions of Pennsylvanians.
The map was drawn by the state's Legislative Reapportionment Commission, which is made up of the four Democratic and Republican caucus leaders of the House and Senate, and a chair appointed by the Pennsylvania Supreme Court:
Mark Nordenberg, chair
Senate Majority Leader Kim Ward (R., Westmoreland)
Senate Minority Leader Jay Costa (D., Allegheny)
House Majority Leader Kerry Benninghoff (R., Centre)
House Minority Leader Joanna McClinton (D., Philadelphia)
The caucus leaders focused on their own chambers, so the House map was developed first by McClinton and Benninghoff before Nordenberg stepped in to draw lines with the two leaders’ input. The commission voted 3 to 2 to approve the map, with the Democrats joining Nordenberg to approve it and the Republicans opposing.
Benninghoff blasted the map in comments before the vote, calling it an extreme partisan gerrymander that will unfairly benefit Democrats.
“The map before us is nothing short of a danger to our system of government that upends established norms and the emphasis on local control and local voices that Pennsylvanians hold dear,” he said.
Now that the map has been introduced, the state Constitution gives a 30-day window for the commission to make changes or for “any person aggrieved by the preliminary plan … to file exceptions with the commission.” The commission then has 30 days to respond to those challenges and make changes before the map is considered final. That kicks off one more 30-day period for people to appeal the map directly to the state Supreme Court.
Pennsylvania redraws its state legislative districts every decade and has a rich history of partisan gerrymandering — in which the political party that controls the map-making process draws districts to gain or maintain power.
To better understand this map and others, The Inquirer is partnering with the nonpartisan Princeton Gerrymandering Project to analyze the districts.
Here's what we found, starting with the question everyone’s asking: How Democratic or Republican is each seat?
The current map favorsRepublicanswith a85–118split, based on the most recent two presidential elections.There are68 strong Democratic districtsand83 strong Republican districts.The remaining52 districts are more competitive, with each side winning between 45% and 55% of votes. Of those,17 districts lean Democratand35 districts lean Republican.
The proposed new map favorsRepublicanswith a99–104split, based on the most recent two presidential elections.There are70 strong Democratic districtsand82 strong Republican districts.The remaining51 districts are more competitive, with each side winning between 45% and 55% of votes. Of those,29 districts lean Democratand22 districts lean Republican.







Our analysis measures the partisan lean of districts based on the average of the two-party presidential vote shares from 2016, when Donald Trump defeated Hillary Clinton by less than 1% of the vote, and 2020, when Joe Biden beat Trump by slightly over 1%.
That's an imperfect metric, partly because past elections don't always neatly predict future ones, and Pennsylvania voters have a history of ticket-splitting, or casting ballots for candidates from different parties in different races. There are Democrats who win in Republican districts, and vice versa.
To help account for that, the analysis uses 55% as the cut-off for what defines a "strong” or “safe” district. Districts where the presidential victor won 50-55% of the vote are categorized as “leaning” toward that party. The table of recent statewide election results shows how competitive districts can flip for different races and in different years, especially in wave elections like 2018.
So while imperfect, our analysis still gives a pretty good sense of the underlying partisanship in each district. Just don’t treat it as an explicit prediction — especially for competitive seats, in wave elections, and in specific or unusual circumstances.
How red or blue the district is sets the playing field, but candidates, campaigns, the broader national and local political environment, and other factors also matter.
Subscribe to The Philadelphia Inquirer
Our reporting is directly supported by reader subscriptions. If you want more data-driven journalism like this story, please subscribe today
What would happen in a 50-50 election?
Pennsylvania is a very evenly divided state, but what happens in a hypothetical election when the statewide vote is exactly split 50% between the two parties? That's one easy way to test a map: If one party wins a large majority of the seats in a 50-50 election, that's a clear sign the map skews in its direction.
The average statewide vote share from the 2016 and 2020 presidential elections was 50.1% Democratic — reflecting that Biden’s victory was slightly larger than Trump’s. That means it was 0.1 percentage points away from a perfectly 50-50 election. So we simply shifted each district’s votes toward Republicans by 0.1 percentage points to see how many districts each party would win in a 50-50 election, based purely on base partisanship.
The proposed map would create 82 solidly Republican seats and 22 Republican-leaning seats in a 50-50 election. That’s a total of 104 seats, or 51.2% — a “partisan bias” of 1.2%, which favors Republicans less than the current map’s 8% partisan bias. There would be 70 solidly Democratic seats and 29 Democratic-leaning seats.
How uneven is the distribution of voters?
It might sound odd, but if you’re a mapmaker trying to help your party, you don’t want to win districts too handily. Instead, you want to maximize the number of wins by drawing districts that can be safely won by your party — but not so safely that you’re “wasting” votes in a blowout.
To gerrymander, it’s better to win three districts with 60% of the vote than to only win two districts with 90%.
To skew the lines, then, mapmakers have two main methods of gerrymandering. The first is by “packing” the other side’s voters into a small number of districts that they win by a lot, essentially sacrificing those seats. The other method is “cracking” the other side’s voters across multiple districts so they can’t win any of them.
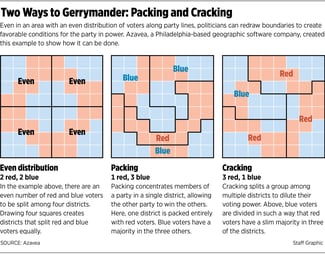
There are several methods to measure maps and identify the kind of skewed vote shares that arise from packing and cracking. Our analysis uses three.
The mean-median difference looks at the difference between a party’s average vote share and median vote share across all the districts. When the difference is large, it means one party is more clustered in its wins than the other party — and potentially being packed and cracked. The mean-median difference in the proposed map is 1.88%, which means Republicans have a slight advantage. The current House map has a difference of 3.28%.
Packed wins, or lopsided wins, is a metric looking at the average margin of Democratic victory and the average margin of Republican victory. If one party’s average margin is much higher, that means its voters are clustered and might signal they’re being intentionally packed. The proposed map’s difference of 1.54% favors Republicans. But compare that with the current map’s 5.45% skew toward Republicans.
So when you look at the presidential vote share for the proposed districts, Democratic seats tend to have either more competitive margins than Republican ones or to be total blowouts with large Democratic margins. Republican seats are more evenly distributed in their margins of victory.
The efficiency gap looks at each district’s “wasted votes” — all the votes cast for the losing candidate, as well as the votes cast for the winner that go beyond the 50% mark needed to win — to see how efficiently both parties’ votes translate to winning seats. The higher the efficiency gap, the more tilted the map is toward one party. The proposed map’s efficiency gap of 1.98% skews slightly toward Republicans. The current map, by comparison, has a pro-Republican efficiency gap of 8.23%.
Are the districts sprawling or compact?
Sprawling districts with long tentacles and weird edges can be a sign that district lines were drawn to carefully include some voters and exclude others for partisan reasons.
There are several ways to measure compactness from a zero to 100 scale. Our analysis includes multiple metrics but we'll focus on just one here, called the Reock score.
Because a circle is the most compact shape, this method draws a circle around each district and looks at how much of the circle is covered by the district. The more compact the shape, the more circular it is, and the closer to 100% of the circle it would cover.
Consider Pennsylvania’s most sprawling and compact districts from the current House map:


We use the average of all the districts’ scores. And because compactness is based only on the shape of districts, not how big or small they are, it’s possible to use the current map for context.
The proposed map has an average Reock score of 42. That means the districts are more compact than the current map, which averages 38.


What's the racial breakdown of each district?
How communities of color are represented is a major legal and political issue, and federal voting rights law tries to protect the ability of those voters to elect the candidates of their choice. Determining whether a map violates the Voting Rights Act is complicated — for example, the common belief that it requires “majority-minority” districts isn't necessarily true and depends in part on racial voting patterns.
Racial and ethnic identity are also complex. The Department of Justice uses multiple means of categorizing people when it analyzes maps for compliance with voting laws.
Here’s a breakdown of each district with non-overlapping categories of 2020 Census data. All Hispanic residents are categorized together regardless of race, and all other categories are for non-Hispanic residents of a single race. This also combines Asian, Native Hawaiian, and Pacific Islander groups into one broad AAPI category, and includes American Indian respondents with those who selected “some other race” or multiple races.
But that’s not the only way people identify. Here’s a breakdown in which people can be categorized in multiple groups. Someone who is Black and Latino, for example, will fall into both the Black and Hispanic or Latino categories.
How do Democrats and Republicans naturally cluster across Pennsylvania?
There’s an important caveat to keep in mind when analyzing any political map of Pennsylvania: Even though the state’s voters are about equally divided between Democrats and Republicans, they aren't evenly distributed.
Cities tend to be heavily packed with Democrats, suburbs get more and more purple the further out from the city, and rural areas are dominated by Republicans. So in densely populated Southeastern Pennsylvania, for example, Philadelphia and its suburbs make up a huge chunk of Democratic votes.
Here’s how that looked in the 2020 election, with one blue dot for every 10 Biden votes and one red dot for every 10 Trump votes:
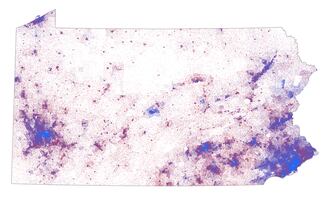
Pennsylvania’s political geography, then, inherently favors Republicans to some extent, because Democrats cluster in cities and dense suburbs.
The question is how much the state’s political geography favors Republicans: What’s the natural range of blue and red seats?
Our partners at the Princeton Gerrymandering Project had computers draw one million maps to find out.
Given a starting map and a set of limitations — such as an acceptable range for the population of each district — they had computers randomly draw maps over and over, measuring the partisan composition of each one and counting the number of blue and red districts.
What emerges is a clear picture of the natural range of safe and competitive seats.
Out of one million computer-drawn maps, the base partisanship of the districts almost always favored Republicans. Pennsylvania’s political geography, sliced into 203 districts, naturally supports about 93 Democratic seats and 110 Republican seats, with very little overlap between the two parties’ ranges of seats.
Of course, some seats are safe, while others are more competitive. Using the two-party vote share based on the average 2016 and 2020 presidential vote, the most common outcomes were between 71 and 75 “safe” seats that are at least 55% Democratic and 18 and 22 seats that lean Democratic with 50-55% of the vote.
Specifically, the single most common Democratic outcome was 73 safe Democratic seats and 20 lean-Democratic seats, which occurred 3.09% of the time. That was followed by 73 safe and 19 lean seats (3.07% of the time), 72 safe and 20 lean (2.88%), and 72 safe and 21 lean (2.85%).
Republicans, on the other hand, naturally have more safe seats, between 89 and 94, along with 17 to 21 seats that lean Republican.
The single most common outcome for Republicans was to have 91 safe Republican seats and 20 Republican-leaning seats, which occurred in 3.17% of the computer-drawn maps. That was followed by 92 safe and 18 lean seats (2.85%), 91 safe and 19 lean (2.79%), and 91 safe and 18 lean (2.76%).
So Pennsylvania’s underlying political geography does help Republicans. Keep that in mind when considering the various metrics for how skewed or fair a map is, and remember: The base partisanship only tells you so much.
Factors like incumbency, the individual candidates and campaigns, the national and local political climate, and access to the ballot box matter, too.
About this story
This article is part of a partnership between The Inquirer and the nonpartisan Princeton Gerrymandering Project to use computational methods to analyze and contextualize political maps. PGP is providing custom data analysis The Inquirer is using in its redistricting coverage. The Inquirer maintains full editorial control of its coverage.
The partisan metrics in this article are based on the average of the two-party 2016 and 2020 presidential vote. Other statewide races were also examined to ensure those scores make sense: U.S. Senate in 2016 and 2018, governor in 2018, and down-ballot statewide row offices in 2020. Results are aggregated into districts from certified precinct results.
Districts are categorized into four groups: “Strong” or “safe” Democratic seats, Democratic-leaning seats, Republican-leaning seats, and “strong" or “safe” Republican seats. We used two rules to categorize districts. First, districts that are won by 55% or more of the presidential vote average are categorized as “strong” seats for the winning party, while districts with victories between 50 and 55% are classified as lean seats. We then use the results of the two most recent U.S. Senate races — from 2016, when Republican Pat Toomey won, and 2018, when Democrat Bob Casey won — to reclassify any “strong” seats as a “lean” district if its voters chose the other party’s Senate candidate in either election.
Staff Contributors
- Reporting: Jonathan Lai, Aseem Shukla
- Design and Development: Sam Morris
- Editing: Dan Hirschhorn
- Data analysis: Princeton Gerrymandering Project